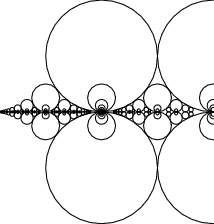
Pick any two integers h and k, then the circle C(h,k) of radius ![]() centered at
centered at ![]() is known as a Ford circle. No matter what and how many hs and ks are picked, none of the Ford circles intersect (and all are tangent to the x-axis). This can be seen by examining the squared distance between the centers of the circles with (h, k) and
is known as a Ford circle. No matter what and how many hs and ks are picked, none of the Ford circles intersect (and all are tangent to the x-axis). This can be seen by examining the squared distance between the centers of the circles with (h, k) and ![]() ,
,
| (1) |
Let s be the sum of the radii
| (2) |
then
| (3) |
But ![]() ,
,![]() and the distance between circle centers is
and the distance between circle centers is ![]() the sum of the circle radii, with equality (and therefore tangency) iff
the sum of the circle radii, with equality (and therefore tangency) iff ![]() .
.
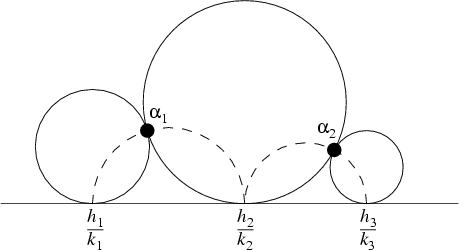
If ![]() ,
,![]() ,
,![]() are three consecutive terms in a Farey sequence, then the circles
are three consecutive terms in a Farey sequence, then the circles ![]() and
and ![]() are tangent at
are tangent at
| (4) |
and the circles ![]() and
and ![]() intersect in
intersect in
| (5) |
Moreover, ![]() lies on the circumference of the semicircle with diameter
lies on the circumference of the semicircle with diameter ![]() and
and ![]() lies on the circumference of the semicircle with diameter
lies on the circumference of the semicircle with diameter ![]() (Apostol 1997, p. 101).
(Apostol 1997, p. 101).
![]()
Adjacent Fraction, Apollonian Gasket, Farey Sequence, Stern-Brocot Tree
![]()
![]()
Apostol, T. M. "Ford Circles." §5.5 in Modular Functions and Dirichlet Series in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 99-102, 1997.
Conway, J. H. and Guy, R. K. "Farey Fractions and Ford Circles." The Book of Numbers. New York: Springer-Verlag, pp. 152-154, 1996.
Ford, L. R. "Fractions." Amer. Math. Monthly 45, 586-601, 1938.
Pickover, C. A. "Fractal Milkshakes and Infinite Archery." Ch. 14 in Keys to Infinity. New York: W. H. Freeman, pp. 117-125, 1995.
Rademacher, H. Higher Mathematics from an Elementary Point of View. Boston, MA: Birkhäuser, 1983.